Computationally Augmented Retrosynthesis: Total Synthesis of Paspaline A and Emindole PB

Daria E. Kim, Joshua E. Zweig and Timothy R. Newhouse (2018)

Highlighted by Jan Jensen

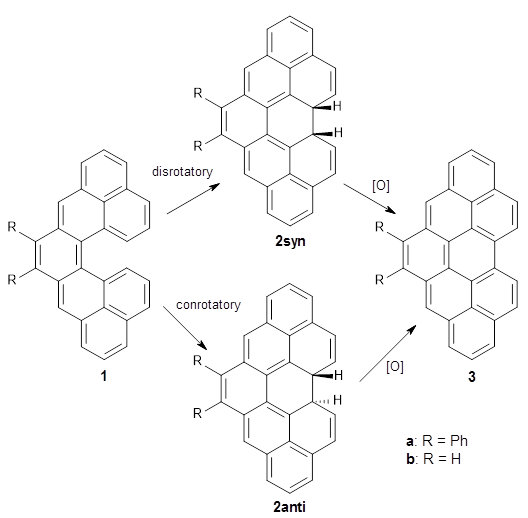
Figure 2 from the paper reproduced under the CC-BY-NC-ND licence

This paper presents a rare example of using quantum chemical TS calculations to guide, rather than post-rationalise, organic synthesis. The authors wanted to design a retrosynthetic path that could be used to make two related natural products, paspaline A and emindole PB, that require either a ring closure (paspaline A) or a methyl shift (emindole PB). Three different routes were possible that lead to different functionalities that were relatively distant from the ring closure/methyl shift, which made it hard to predict the best route by chemical intuition.

Instead the authors used mPW1PW91/6-31+G(d,p)//B3LYP/6-31G(d) to find the TSs for both reactions for each of the three routes to predict the best route, which turns out to be "C". Route C did indeed work great in practice, while route A (predicted to be worst route) didn't give the desired results.

My guess is that the key here is that the synthetic question was reduced to a question of relative barrier heights of closely related reactions, i.e. ΔΔΔG^‡ = ΔΔG^‡(4→5) - ΔΔG^‡(4→6), which leads to maximum error cancellation. I hope this paper will lead to more use of QM to guide synthetic decisions and more work on making TS calculations even more accessible to synthetic chemists


This work is licensed under a Creative Commons Attribution 4.0 International License.

Electrocyclic reactions of cethrene derivatives

Šolomek, T.; Ravat, P.; Mou, Z.; Kertesz, M.; Juríček, M., "Cethrene: The Chameleon of Woodward–Hoffmann Rules." J. Org. Chem. 2018, 83, 4769-4774
Ravat, P.; Šolomek, T.; Häussinger, D.; Blacque, O.; Juríček, M., "Dimethylcethrene: A Chiroptical Diradicaloid Photoswitch." J. Am. Chem. Soc. 2018, 140, 10839-10847.
Contributed by Steven Bacharach
Reposted from Computational Organic Chemistry with permission

Pericyclic reactions remain a fruitful area of research despite the seminal publication of the Woodward-Hoffmann rules decades ago. Here are two related papers of pericyclic reactions that violate the Woodward-Hoffmann rules.

First, Solomek, Ravat, Mou, Kertesz, and Jurícek reported on the thermal and photochemical electrocyclization reaction of diphenylcetherene 1a.¹ Though they were not able to directly detect the intermediate 2, through careful examination of the photochemical reaction, they were able to infer that the thermal cyclization goes via the formally forbidden conrotatory pathway (see Scheme 1).

Scheme 2.

Kinetic studies estimate the activation barrier is 14.1 kcal mol^-1. They performed DFT computations of the parent 1b using a variety of functionals with both restricted and unrestricted wavefunctions. The allowed pathway to 2syn is predicted to be greater than 27 kcal mol^-1, while the formally forbidden pathway to 2anti is estimated to have a lower barrier of about 23 kcal mol^-1. The two transition states for these different pathways are shown in Figure 1, and the sterics that force a helical structure to 1 help make the forbidden pathway more favorable.

TS(1b→2b-syn)

TS(1b→2b-anti)

Figure 1. (U)B3LYP/6-31G optimized geometries of the transition states taking 1 into 2.

Nonetheless, all of the DFT computations significantly overestimate the activation barrier. The authors make the case that a low-lying singlet excited state results in an early conical intersection that reduces the symmetry from C₂ to C₁. In this lower symmetry pathway, all of the states can mix, leading to a lower barrier. However, since DFT is intrinsically a single Slater configuration, the mixing of the other states is not accounted for, leading to the overestimated barrier height.

In a follow up study, this group examined the thermal and photo cyclization of 13,14-dimethylcethrene 4.² The added methyl groups make the centhrene backbone more helical, and this precludes the formal allowed disrotatory process. The methyl groups also prohibit the oxidation that occurs with 1, driven by aromatization, allowing for the isolation of the direct product of the cyclization 5. This antistereochemistry is confirmed by NMR and x-ray crystallography. The interconversion between 4 and 5 can be controlled by heat and light, making the system an interesting photoswitch.

Also of interest is the singlet-triplet gap of 1 and 4. The DFT computed ΔE_ST is about 10 kcal mol^-1 for 4, larger than the computed value of 6 kcal mol^-1 for 1b. The EPR of 1b does show a signal while that of 4has no signal. To assess the role of the methyl group, they computed the singlet triplet gaps for 1b and 4at two different geometries: where the distance between the carbons bearing the methyl groups is that in 1b (3.03 Å) and in 4 (3.37 Å). The lengthening of this distance by the methyl substituents is due to increased helical twist in 4 than in 1b. For 1b, the gap increases with twisting, from 7.1 to 8.3 kcal mol^-1, while for 4 the gap increases by 1.8 kcal mol^-1 with the increased twisting. This change is less than the effect of methyl substitution, which increases the gap by 2.2 kcal mol^-1 at the shorter distance and 2.8 kcal mol^-1 at the longer distance. Thus, the electronic (orbital) effect of methyl substitution affects the singlet-triplet gap more than the geometric twisting.

References

1) Šolomek, T.; Ravat, P.; Mou, Z.; Kertesz, M.; Juríček, M., "Cethrene: The Chameleon of Woodward–Hoffmann Rules." J. Org. Chem. 2018, 83, 4769-4774, DOI: 10.1021/acs.joc.8b00656.

2) Ravat, P.; Šolomek, T.; Häussinger, D.; Blacque, O.; Juríček, M., "Dimethylcethrene: A Chiroptical Diradicaloid Photoswitch." J. Am. Chem. Soc. 2018, 140, 10839-10847, DOI: 10.1021/jacs.8b05465.

InChIs

1b: InChI=1S/C28H16/c1-5-17-7-3-11-23-25(17)19(9-1)15-21-13-14-22-16-20-10-2-6-18-8-4-12-24(26(18)20)28(22)27(21)23/h1-16H
InChIKey=GBMHAGKZRAVBDO-UHFFFAOYSA-N

4: InChI=1S/C30H20/c1-17-9-11-19-5-3-7-21-15-23-13-14-24-16-22-8-4-6-20-12-10-18(2)26(28(20)22)30(24)29(23)25(17)27(19)21/h3-16H,1-2H3
InChIKey=MXTVFWTUCPRNIW-UHFFFAOYSA-N

5: nChI=1S/C30H20/c1-29-13-11-17-5-3-7-19-15-21-9-10-22-16-20-8-4-6-18-12-14-30(29,2)28(24(18)20)26(22)25(21)27(29)23(17)19/h3-16H,1-2H3/t29-,30-/m0/s1
InChIKey=SUMMGEBJORQMAI-KYJUHHDHSA-N

'
This work is licensed under a Creative Commons Attribution-NoDerivs 3.0 Unported License.