London Dispersion Enables the Shortest Intermolecular Hydrocarbon H···H Contact.

Rösel, S.; Quanz, H.; Logemann, C.; Becker, J.; Mossou, E.; Cañadillas-Delgado, L.; Caldeweyher, E.; Grimme, S.; Schreiner, P. R.,  J. Am. Chem. Soc. 2017, 139, 7428–7431
Contributed by Steven Bacharach
Reposted from Computational Organic Chemistry with permission

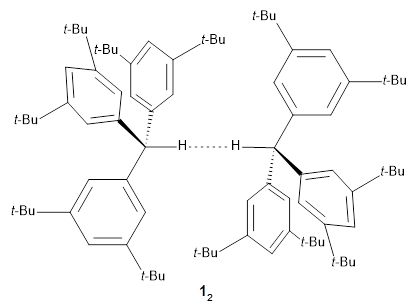
Following on previous work (see these posts on ladderane and hexaphenylethane), Schreiner, Grimme and co-workers have examined the structure of the all-meta tri(di-t-butylphenyl)methane dimer 1₂.¹ In the study of hexaphenylethane,² Schreiner and Grimme note that t-butyl groups stabilize highly congested structures through dispersion, identifying them as “dispersion energy donors”.³ The idea here is that the dimer of 1 will be stabilized by these many t-butyl groups. In fact, the neutron diffraction study of the crystal structure of 1₂ shows an extremely close approach of the two methane hydrogens of only 1.566 Å, the record holder for the closest approach of two formally non-bonding hydrogen atoms.

To understand the nature of this dimeric structure, they employed a variety of computational techniques. (Shown in Figure 1 is the B3LYPD3^ATM(BJ)/def2-TZVPP optimized geometry of 1₂.) The HSE-3c (a DFT composite method) optimized crystal structure predicts the H^…H distance is 1.555 Å. The computed gas phase structure lengthens the distance to 1.634 Å, indicating a small, but essential, role for packing forces. Energy decomposition analysis of 1₂ at B3LYP-D3^ATM(BJ)/def2-TZVPP indicates a dominant role for dispersion in holding the dimer together. While 1₂ is bound by about 8 kcal mol^-1, the analogue of 1₂lacking all of the t-butyl groups (the dimer of triphenylmethane 2₂) is unbound by over 8 kcal mol^-1. Topological electron density analysis does show a bond critical point between the two formally unbound hydrogen atoms, and the noncovalent interaction plot shows an attractive region between these two atoms.

Figure 1. ATM(BJ)/def2-TZVPP optimized geometry of 1₂, with most of the hydrogens suppressed for clarity. (Selecting the molecule will launch Jmol with the full structure, including the hydrogens.)

References

1) Rösel, S.; Quanz, H.; Logemann, C.; Becker, J.; Mossou, E.; Cañadillas-Delgado, L.; Caldeweyher, E.; Grimme, S.; Schreiner, P. R., "London Dispersion Enables the Shortest Intermolecular Hydrocarbon H···H Contact." J. Am. Chem. Soc. 2017, 139, 7428–7431, DOI: 10.1021/jacs.7b01879.

2) Grimme, S.; Schreiner, P. R., "Steric Crowding Can Stabilize a Labile Molecule: Solving the Hexaphenylethane Riddle." Angew. Chem. Int. Ed. 2011, 50 (52), 12639-12642, DOI: 10.1002/anie.201103615.

3) Grimme, S.; Huenerbein, R.; Ehrlich, S., "On the Importance of the Dispersion Energy for the Thermodynamic Stability of Molecules." ChemPhysChem 2011, 12 (7), 1258-1261, DOI: 10.1002/cphc.201100127.

InChIs

1: InChI=1S/C43H64/c1-38(2,3)31-19-28(20-32(25-31)39(4,5)6)37(29-21-33(40(7,8)9)26-34(22-29)41(10,11)12)30-23-35(42(13,14)15)27-36(24-30)43(16,17)18/h19-27,37H,1-18H3
InChIKey=VFNQDWKFTWSJAU-UHFFFAOYSA-N

'
This work is licensed under a Creative Commons Attribution-NoDerivs 3.0 Unported License.