A Deep Neural Network with Minimal Chemistry Knowledge Matches the Performance of Expert-developed QSAR/QSPR Models

Garrett B. Goh, Charles Siegel, Abhinav Vishnu, Nathan O. Hodas, Nathan Baker (2017)

Highlighted by Jan Jensen

Figure 1: The key difference in using deep learning algorithms as a machine learning tool as opposed to a “machine intelligence” tool is the assistance, augmentation and possible replacement, for human-led tasks like feature engineering in computational chemistry.

A lot of machine learning research in chemistry is focussed on finding the best descriptors for the property of interest.  This paper shows that simply using 2D images of molecules leads to similarly accurate predictions of solvation free energies, in vitro HIV activity, and in vivo toxicity. 

This seems to me an appropriate "null-model" that all machine learning studies should include. Another option would be SMILES strings or some representation thereof. If your fancy descriptor doesn't lead to significantly better predictions then it's back to the drawing board.

The manuscript doesn't mention code availability but one of the co-authors tells me that they plan to make to code available when it is ready.

This work is licensed under a Creative Commons Attribution 4.0 International License.

London Dispersion Enables the Shortest Intermolecular Hydrocarbon H···H Contact.

Rösel, S.; Quanz, H.; Logemann, C.; Becker, J.; Mossou, E.; Cañadillas-Delgado, L.; Caldeweyher, E.; Grimme, S.; Schreiner, P. R.,  J. Am. Chem. Soc. 2017, 139, 7428–7431
Contributed by Steven Bacharach
Reposted from Computational Organic Chemistry with permission

Following on previous work (see these posts on ladderane and hexaphenylethane), Schreiner, Grimme and co-workers have examined the structure of the all-meta tri(di-t-butylphenyl)methane dimer 1₂.¹ In the study of hexaphenylethane,² Schreiner and Grimme note that t-butyl groups stabilize highly congested structures through dispersion, identifying them as “dispersion energy donors”.³ The idea here is that the dimer of 1 will be stabilized by these many t-butyl groups. In fact, the neutron diffraction study of the crystal structure of 1₂ shows an extremely close approach of the two methane hydrogens of only 1.566 Å, the record holder for the closest approach of two formally non-bonding hydrogen atoms.

To understand the nature of this dimeric structure, they employed a variety of computational techniques. (Shown in Figure 1 is the B3LYPD3^ATM(BJ)/def2-TZVPP optimized geometry of 1₂.) The HSE-3c (a DFT composite method) optimized crystal structure predicts the H^…H distance is 1.555 Å. The computed gas phase structure lengthens the distance to 1.634 Å, indicating a small, but essential, role for packing forces. Energy decomposition analysis of 1₂ at B3LYP-D3^ATM(BJ)/def2-TZVPP indicates a dominant role for dispersion in holding the dimer together. While 1₂ is bound by about 8 kcal mol^-1, the analogue of 1₂lacking all of the t-butyl groups (the dimer of triphenylmethane 2₂) is unbound by over 8 kcal mol^-1. Topological electron density analysis does show a bond critical point between the two formally unbound hydrogen atoms, and the noncovalent interaction plot shows an attractive region between these two atoms.

Figure 1. ATM(BJ)/def2-TZVPP optimized geometry of 1₂, with most of the hydrogens suppressed for clarity. (Selecting the molecule will launch Jmol with the full structure, including the hydrogens.)

References

1) Rösel, S.; Quanz, H.; Logemann, C.; Becker, J.; Mossou, E.; Cañadillas-Delgado, L.; Caldeweyher, E.; Grimme, S.; Schreiner, P. R., "London Dispersion Enables the Shortest Intermolecular Hydrocarbon H···H Contact." J. Am. Chem. Soc. 2017, 139, 7428–7431, DOI: 10.1021/jacs.7b01879.

2) Grimme, S.; Schreiner, P. R., "Steric Crowding Can Stabilize a Labile Molecule: Solving the Hexaphenylethane Riddle." Angew. Chem. Int. Ed. 2011, 50 (52), 12639-12642, DOI: 10.1002/anie.201103615.

3) Grimme, S.; Huenerbein, R.; Ehrlich, S., "On the Importance of the Dispersion Energy for the Thermodynamic Stability of Molecules." ChemPhysChem 2011, 12 (7), 1258-1261, DOI: 10.1002/cphc.201100127.

InChIs

1: InChI=1S/C43H64/c1-38(2,3)31-19-28(20-32(25-31)39(4,5)6)37(29-21-33(40(7,8)9)26-34(22-29)41(10,11)12)30-23-35(42(13,14)15)27-36(24-30)43(16,17)18/h19-27,37H,1-18H3
InChIKey=VFNQDWKFTWSJAU-UHFFFAOYSA-N

'
This work is licensed under a Creative Commons Attribution-NoDerivs 3.0 Unported License.

Dynamic Effects Responsible for High Selectivity in a [3,3] Sigmatropic Rearrangement Featuring a Bispericyclic Transition State

Villar López, R.; Faza, O. N.; Silva López, C., J. Org. Chem. 2017, 82 (9), 4758-4765

Contributed by Steven Bacharach

Reposted from Computational Organic Chemistry with permission

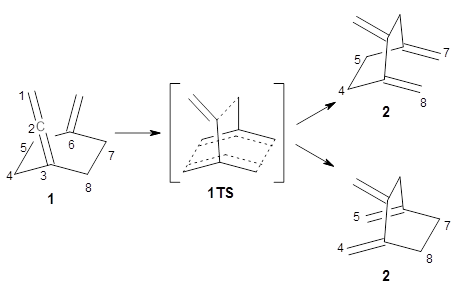
Bispericyclic reactions occur when two different pericyclic reactions merge to have a single transition state. An example of this is the joining of two [3,3]-sigmatopic rearrangements of 1 that merge to have a single transition state. Lopez, Faza, and Lopez have examined the dynamics of this reaction.¹

Because of the symmetry of the species along this reaction pathway, the products of the two different rearrangements are identical, and will be formed in equal amounts, though they are produced from a single transition state with the reaction pathway bifurcating due to a valley-ridge inflection post TS.

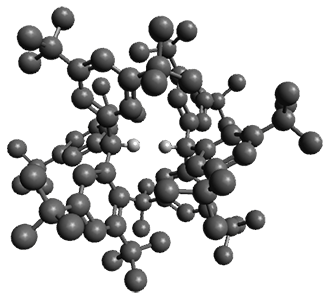
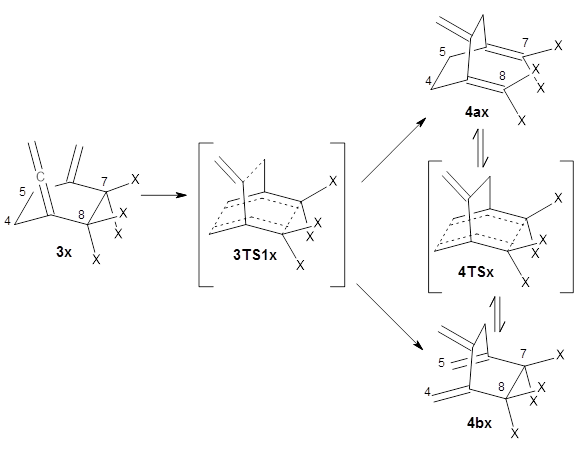
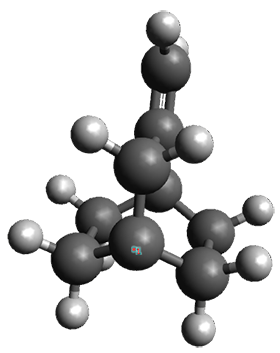
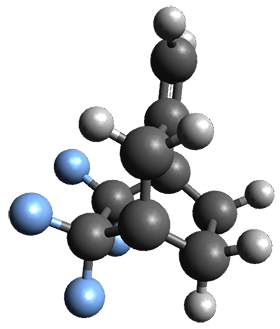
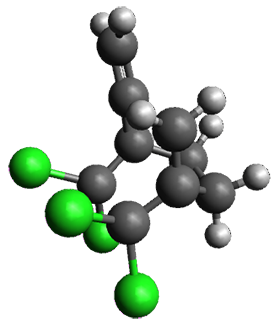
The interesting twist that is explored here is when 1 is substituted in order to break the symmetry. The authors have examined 3x with either fluorine, chlorine, or bromine. The critical points on the reactions surface were optimized at M06-2X/Def2TZVPP. In all three cases a single bispericyclic transition state 3TS1x is found, which leads to products 4a and 4b. A second transition state 4TSx corresponds to the [3,3]-rearrangement that interconverts the two products. The structures of 1TS, 3TS1F, and 3TS1Cl are shown in Figure 1.

1TS

3TS1F

3TS1Cl

Figure 1. M06-2X/Def2TZVPP optimized geometries of 1TS, 3TS1F, and 3TS1Cl.

The halogen substitution breaks the symmetry of the reaction path. This leads to a number of important changes. First, the C₄-C₅ and C₇-C₈ distances, which are identical in 1TS, are different in the halogen cases. Interestingly, the distortions are dependent on the halogen: in 3TS1F C₄-C₅ is 0.2 Å longer than C₇-C₈, but in 3TS1Cl C₇-C₈ is much longer (by 0.65 Å) than C₄-C₅. Second, the products are no longer equivalent with the halogen substitution. Again, this is halogen dependent: 4bF is 4.0 kcal mol^-1 lower in energy than 4aF, while 4aCl is 8.2 kcal mol^-1 lower than 4bCl.

These difference manifest in very different reaction dynamics. With trajectories initiated at the first (bispericyclic) transiting state, 89% end at 4bF and 9% end at 4aF, a ratio far from unity that might be expected from both products resulting from passage through the same TS. The situation is even more extreme for the chlorine case, where all 200 trajectories end in 4aCl. This is yet another example of the role that dynamics play in reaction outcomes (see these many previous posts).

References

1) Villar López, R.; Faza, O. N.; Silva López, C., "Dynamic Effects Responsible for High Selectivity in a [3,3] Sigmatropic Rearrangement Featuring a Bispericyclic Transition State." J. Org. Chem. 2017, 82 (9), 4758-4765, DOI: 10.1021/acs.joc.7b00425.

InChIs

1: InChI=1S/C9H12/c1-3-9-6-4-8(2)5-7-9/h1-2,4-7H2
InChIKey=RRXCPJIEZVQPSZ-UHFFFAOYSA-N

2: InChI<=1S/C9H12/c1-7-4-5-8(2)9(3)6-7/h1-6H2
InChIKey=AMBNQWVPTPHADI-UHFFFAOYSA-N

3F: InChI=1S/C9H8F4/c1-3-7-5-4-6(2)8(10,11)9(7,12)13/h1-2,4-5H2
InChIKey=VZFAQFJKHDWJDN-UHFFFAOYSA-N

3Cl: InChI=1S/C9H8Cl4/c1-3-7-5-4-6(2)8(10,11)9(7,12)13/h1-2,4-5H2
InChIKey=AIVUHFMHIMNOJB-UHFFFAOYSA-N

4aF: InChI=1S/C9H8F4/c1-5-4-6(8(10)11)2-3-7(5)9(12)13/h1-4H2
InChIKey=NAUUHIHYMAOMIF-UHFFFAOYSA-N

4aCl: InChI=1S/C9H8Cl4/c1-5-4-6(8(10)11)2-3-7(5)9(12)13/h1-4H2
InChIKey=MMCKDJXQYSGQEH-UHFFFAOYSA-N

4bF: InChI=1S/C9H8F4/c1-5-4-6(2)8(10,11)9(12,13)7(5)3/h1-4H2
InChIKey=LMFNAIRCNARWSX-UHFFFAOYSA-N

4bCl: InChI=1S/C9H8Cl4/c1-5-4-6(2)8(10,11)9(12,13)7(5)3/h1-4H2
InChIKey=NOFFASDSCUGRTP-UHFFFAOYSA-N

'
This work is licensed under a Creative Commons Attribution-NoDerivs 3.0 Unported License.